Water vapor transmission is a rather confusing issue. The difference in vapor pressure between two sides of a building envelope assembly is the driving force behind vapor transmission. (Although by comparison, gaps in the envelope account for vastly greater amounts moisture migration due to vapor-laden air infiltration.)

 

Vapor = Latent Heat; Air Temperature = Sensible Heat

The vapor pressure differential is to latent heat flow as the dry-bulb air temperature is to sensible heat flow. Just as sensible heat always wants to approach equilibrium in an environment, so too does latent heat. If there is less vapor pressure on the other side of a wall, vapor will slowly "drive" through the wall toward the other side. Very similar to how thermal energy (heat) will slowly drive through the wall if the other side is cooler.

Just as various building materials will resist the conductive transfer of heat at different rates, they will also resist the diffusion of water vapor at different rates as well.

There are two measures used to gauge the rate of water vapor transmission through building materials: permeance and permeability.

 

Permeance

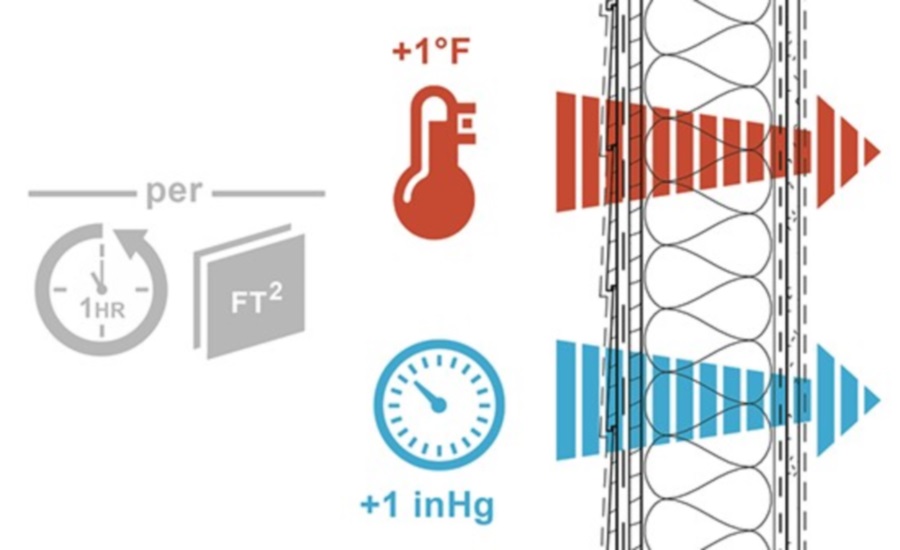
The permeance of a building material is the latent heat equivalent of sensible heat conductance. Permeance indicates that water vapor transmission rate over the course of one hour through one square foot of a material of a given thickness at a specified vapor pressure, expressed in perms (gr/hr●ft2●inHg).

As clarified in a study published by the University of Alaska Fairbanks (UAF) Cooperative Extension Service:

If a material has a perm rating of 1.0, we know that in 1 hour, when the vapor pressure difference between the cold side and the warm side of the material is equal to 1-inch of mercury (1-inch Hg), 1 grain of water vapor will pass through 1-square-foot of the material. One grain of water is equal to 1/7,000 of a pound.

"Inch of mercury" (inHg or in. Hg or Hg) is a unit of measurement for vapor pressure. Historically, it has been utilized to gauge barometric pressure in weather reports.

 

Permeability

The permeability of a building material is the latent heat equivalent of sensible heat conductivity.

Permeability can be thought of as permeance per unit of thickness—or perm-inches. This becomes important when considering building materials that come in a variety of thicknesses within an assembly, such as insulation.

Be careful to not confuse perms and perm-inches. Also, always be mindful of whether imperial units (I-P) or the International System of Units (SI) are being utilized.

 

What constitutes vapor permeable?

The less permeable a building material is, the greater its resistance to water vapor transmission. A vapor retarder is essentially any building material that exhibits a very low permeance (very high resistance to water vapor transmission).

Table by Daniel Overbey.
 

What are vapor retarders?

The specific location of envelope components that serve to impede water vapor diffusion (commonly referred to as vapor retarders) will vary with envelope component/orientation, construction types, and climate. The basic principle is for the retarder to drastically reduce the flow of water vapor before it can come into contact with its dew point temperature within the assembly. Note that is almost impossible to fully stop vapor diffusion, thus the once common term vapor barrier is a misnomer and should not be used.

The International Residential Code (IRC) categories vapor retarders based on their permeance (as defined above):

  • Class 1: vapor impermeable
  • Class 2: vapor semi-impermeable
  • Class 3: vapor semi-permeable

Before determining the selection and placement of a vapor retarder, check for any climate-specific requirements for the climate zone in which a project is located.

 

How to determine the permeance of building materials

The permeance of building materials is determined by testing the materials in accordance with ASTM E96 / E96M-15, Standard Test Methods for Water Vapor Transmission of Materials.

ASTM E96 identifies two methods to determine the water vapor transmission of a material: desiccant (dry cup) and water (wet cup). These two test methods are similar in setup but the service conditions are different and the results are not incomparable. The dry cup test is designed to simulate a heated dry building during a pouring rain, measuring the moisture drive through the building assembly. The wet cup test measures the vapor drive moving in the opposite direction.

Table by Daniel Overbey.
 

How to calculate the total resistance to water vapor transmission in a building envelope assembly?

When we define a material's R-value, we typically assume that it is in reference to the resistance to conductive heat flow (which is the inverse of the thermal transmission rate, or U-factor).

However, we can describe a material's resistance to water vapor transmission in a similar sense. It's the other R-value. Therefore, it is appropriate to distinguish between the two:

  • Rt-value is for resistance to thermal energy (i.e., heat) transmission.
  • Rp-value is for resistance to water vapor transmission, which is the inverse of the permeance rating (1/perm).

One may calculate Rp-values just like Rt-values for an assembly.

When calculating vapor permeance of multiple layers of an entire building envelope assembly (e.g., a wall or roof), take the following steps (and see the example below):

1. Find the perm rating for every component.

First, determine the permeance of every constituent component. (In order to determine the perm value where perm-inches are used, simply divide the tabulated value by the material's actual thickness in inches).

2. Calculate the reciprocal of every perm rating.

Take the reciprocal of every component's permeance value (1/perm) in order to determine their respective Rp-values.

3. Add up the Rp-values.

Then, one may add up all of the Rp-values just as one would add up Rt-values. Now, you have the total ΣRp-value for the assembly. The higher an R-value, the greater the resistance.

4. Take the reciprocal of the total Rp-value.

Take the reciprocal (1/ΣRp-value) to determine the permeance of the entire building envelope assembly.

Please note, it would be erroneous to simply add up the permeance of every constituent assembly material. According to the UAF report:

The perm ratings given are for stated thicknesses of materials. Generally, doubling material thickness halves water vapor transmission: if 1-inch of a material has a perm rating of 2.0, then for 2 inches, the perm rating would be 1.0. With paints, however, adding a second coat more than halves the water vapor transmission.

As with determining the total U-factor of an assembly, one must add up all to the Rt-values and then take the reciprocal in order to determine the permeance of the entire building envelope assembly.

Table and illustration by Daniel Overbey.